The gravity field in Iceland

and the foundations of the land

 

Research results 1988-1992

In the years around 1990 we, Stefán G. Magnússon and Freyr Þórarinsson, carried out various kinds of research on gravitational field and magnetic fields, working both on developing research methods and on the processing of Icelandic data. The data was obtained from the National Energy Authority and the Science Institute of the University of Iceland and our co-authors of the articles on the data processing were from those institutions.

The first phase of our research focused on developing methods to analyse directionality in maps of gravitational and magnetic fields and topography. To do this, we wrote computer programs that used two-dimensional Fourier transforms to turn a map into a directional power spectrum. We wrote papers about this work, e.g. a paper in Geophysics. Another paper appeared in Jökull a year later, where these methods were applied to data from Southwest Iceland.

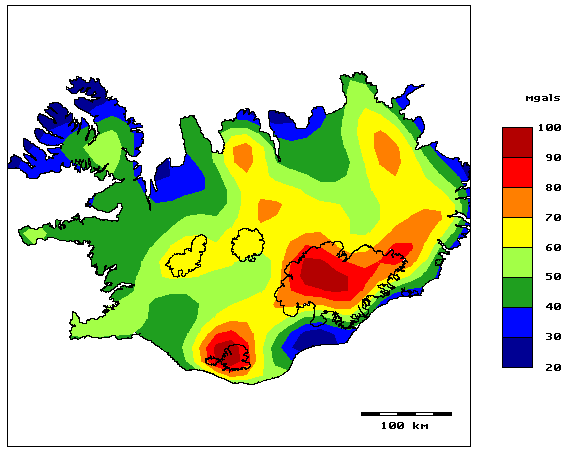
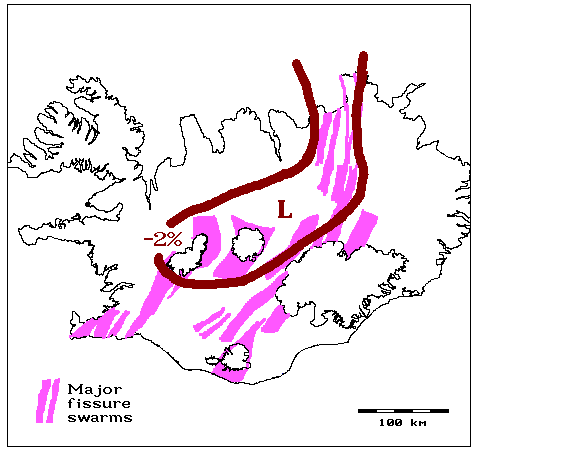
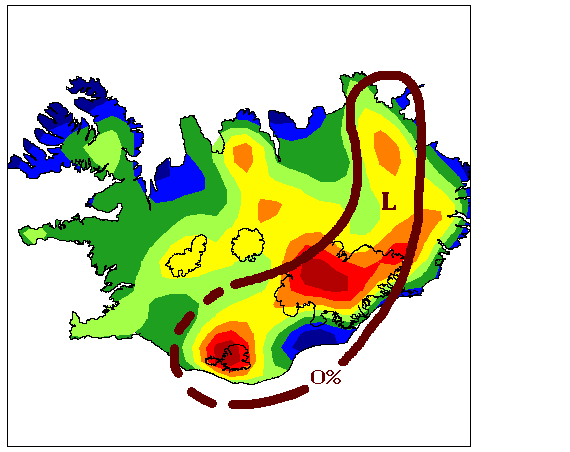
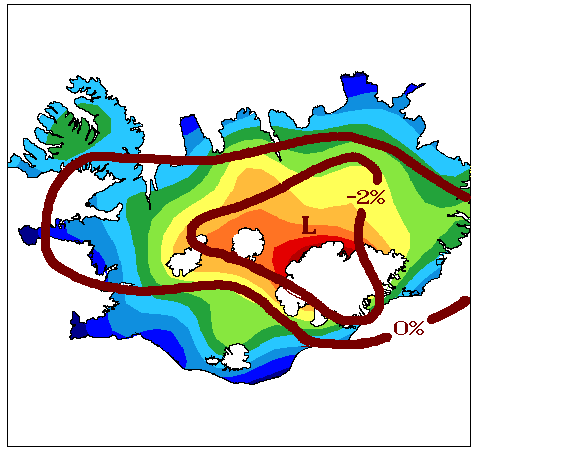
One of the things to consider in processing gravity data is the choice of Bouguer density used correct the effects of landscape in the measurements. A common approach to this is to minimize the correlation between landscape and corrected gravity field (often referred to as Nettleton's method), but we soon realized that this method was not always suitable for Icelandic data. The reason is that this method assumes the landscape to be resting on a rigid inflexible substrate, while in Iceland the crust is thinner and more flexible than on the continents where Nettelton's method was developed. So we developed another method based on minimizing the fractional width of the corrected gravitational field and published it in a paper in Geophysics.

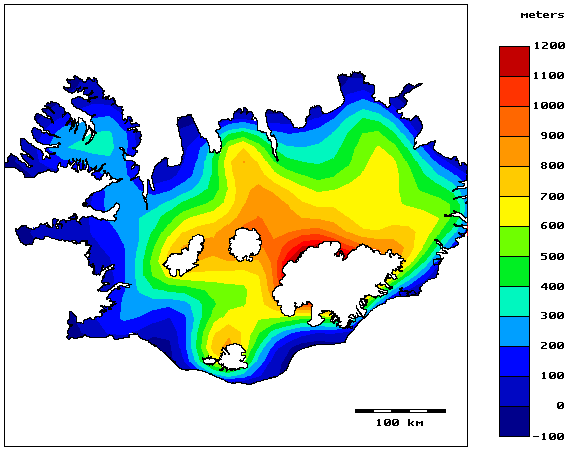
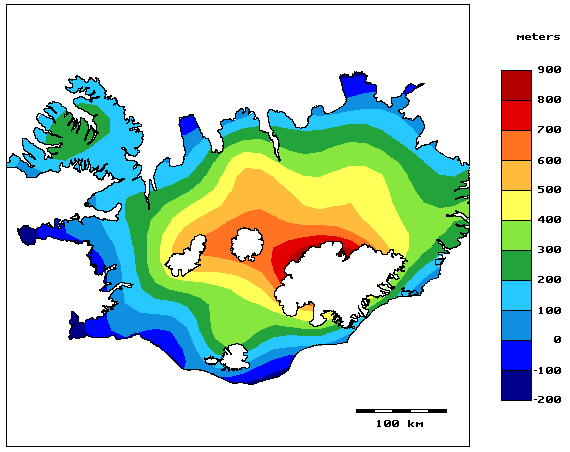
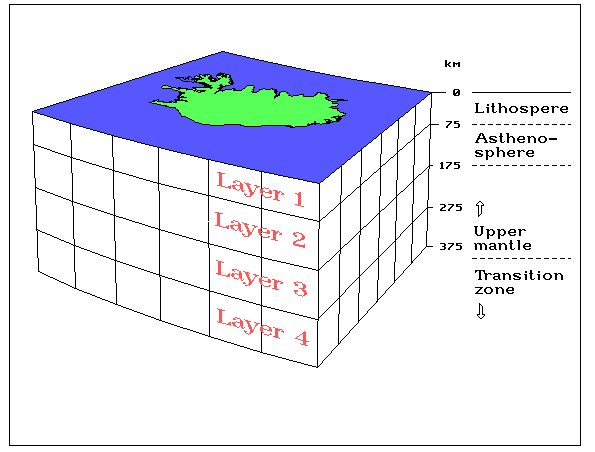
The research on suitable Bouguer density focused our attention on the load-bearing capacity of the Icelandic earth's crust and the boundary between rigid landscapes and floating, and the main findings of our research in this area are discussed alongside figures 1-3 below. Then we got acquainted with the paper by Kristján Tryggvason et al. showing a seismic image of the hot spot beneath Iceland, and found that what was shown there seemed to be in good agreement with our research (figures 5-8). These ideas were presented in various ways at several conferences in Iceland and abroad, but before articles about them were published in magazines, both of us had turned to other subjects. However, the idea has survived to give an account of these discussions in an accessible place, and that is why this website has now been created.

More than three decades have passed since the material presented here was created, but no attempt has been made to update it in light of new results. The reader can therefore imagine this to be a message in a bottle that he receives long after its contents were created. Comments on the topic can be sent to freyrth@hotmail.com.

Figure 1. Landscape after low-pass filtering

Think of an iceberg floating in the sea. Because its density is about 9/10 that of the sea, the iceberg floats so that one-tenth of it is above the water, supported by the part that is underwater. It is in buoyant equilibrium and the equilibrium level is at the sea surface. Individual highs and lows on the surface of the iceberg are supported by the rigid ice - it is the iceberg as a whole that is in buoyant balance.

Iceland as a whole is in the same kind of buoyancy balance - beneath the land there is an anomaly in the mantle density that supports it. Individual mountains and valleys are supported by the rigid earth's crust - it is the land as a whole that is in buoyant equilibrium.

The landscape that is supported by a rigid earth's crust, the rigid landscape, is often characterized by a geometric property called a constant fractal dimension.^* The rule that applies to such landscapes is that if the height difference between two places is considered statistically as a function of the distance between them, an increasing standard deviation can be expected with increasing distance. However, a landscape in perfect floating balance, floating landscape, is characterized by a normally distributed height differences and standard deviations that do not increase with increasing distance between places.

Our studies^† showed a constant fractional dimension from, say, one kilometer out to a maximum of 20-30 km, after which the standard deviation of the height difference stopped growing with increasing distance between places. This can be interpreted as showing that the Earth's crust carries landscape features with a diameter of up to 20-30 km, after which buoyancy forces take over the weight.^‡

On these grounds, rigid landscapes are separated from floating landscapes simply by filtering them away, using a low-pass filter with a cut-off frequency corresponding to 40 kilometres. The result is a map of the landscape floating on its substrate (Figure 1).

* A surface with a constant fractional dimension is said to be self-similar at all scales.

† Freyr Þórarinsson & Stefán G. Magnússon, 1990. Bouguer density determination by fractal analysis. Geophysics, vol. 55. no. 7.

‡ The distance over which the Earth's lithosphere flexes or bends due to the loading of surface features is called the flexural wavelength. In Iceland, this distance seems to range from a few kilometres (in active volcanic zones) to a few tens of kilometres.

Figure 2. Free-air gravity data after low-pass filtering

Gravity measurements are made across mountains and valleys at different altitudes above sea level. The Earth's attraction is weaker up in the mountains than in lowlands, due to the increased distance from the center of the Earth. Therefore, gravimetry has to be corrected for measuring point altitude before measured values can be compared. This height correction is called free-air correction.

But the rigid landscape also affects measured gravity values - nearby mountains pull on the instruments and the valleys have a comparable effect. Landscape effects are most commonly corrected by a Bouguer correction (see figure 4), but this effect can also be removed from the measurements by filtration identical to that applied to the landscape in figure 1; low-pass filtering with a cut-off frequency corresponding to 40 kilometres (figure 2).

Back to the iceberg. Imagine gravitational measurements had been made on top of it, measuring only the attraction from the ice and the sea beneath it. When free-air corrections had been made and the gravity values calculated down to the sea level - the equilibrium surface - the result would have been a flat surface; everywhere the same attraction was measured because everywhere there was the same mass beneath the measuring point on top of the iceberg. However, if the elevation adjustment had been based on a surface other than the actual equilibrium surface, the result would not have been a flat surface; some height adjustments would have been overestimates and others underestimated.

Obviously, the gravity values in Figure 2 are not a smooth surface; they span about 70 milligal. The deviations are due to the fact that the free-air correction is based on sea level, but the equilibrium surface is not at sea level. As the equilibrium surface rises, the free-air correction overestimates of the thickness of the floating landscape, and we get a positive deviation in the map in Figure 2.

Figure 3. Landscape in buoyant equilibrium, low-pass filtered

The landscape resting on top of the equilibrium surface can be referred to as a landscape in buoyant equilibrium. If the equilibrium surface followed sea level, this would be the landscape that faced us; outline of the iceberg above the sea surface. Thus, the floating landscape in Figure 1 can be said to be composed of two components:

height of floating landscape	= height of equilibrium surface
	+ thickness of landscape in buoyant equilibrium

By selecting values for the density of the Earth's crust and the gravity field strength at sea level, the gravity anomalies in Figure 2 can be converted into altitude anomalies; converting milligals to meters. The outcome of such calculations is the altitude of the equilibrium surface. When those values are subtracted from the elevation values of the floating landscape (Figure 1), the thickness of the landscape is obtained in buoyant equilibrium; the thickness of the landscape resting on the equilibrium plane.

Gunnar Þorbergsson et al. published gravity data from the National Energy Authority corrected with a Bouguer specific gravity of 2600 kg/m³.^* Trausti Einarsson estimated in his paper that the gravitational field at sea level was about 40 mgal.^† These values are used to calculate the thickness of landscape in buoyant equilibrium; the result is shown in Figure 3.

Here the shape of the floating landlandscape has been greatly simplified from Figure 1 and is essentially as expected: The land rises highest above the centre of the hotspot under the western side of Vatnajökull and the drift of the plate boundary to ESE leaves a trail to the WNW. By tinkering with the Bouguer density used to calculate the height of the equilibrium surface, it would be possible to further sharpen these main features in figure 3, but here the preferred approach is to use these conventional values.

* Gunnar Þorbergsson, Ingi Þór Magnusson & Guðmundur Pálmason 1990. Gravity data and a gravity map of Iceland (in Icelandic). Orkustofnun, 1990.

† Trausti Einarsson 1954. A survey of gravity in Iceland. Soc. Sci. Isl. Rit 30.

Figure 4. Bouguer corrected gravity, low-pass filtered

Once the gravity measurements have been adjusted for height, the effect of the landscape on the measured values must then be corrected. The standard way of doing this is the so-called Bouguer correction, where the impact of the local landscape is calculated using a selected density for the rock and corrected for this effect. It is sometimes described such that the Bouguer values should correspond to the gravitational values that would be measured if the mountains were scraped away. If the landscape were in buoyant equilibrium, the Bouguer map would show the mass anomaly that supported the landscape - a kind of reflection of the landscape.

Figure 4 shows a Bouguer corrected map based on Orkustofnun's gravity measurements, filtered using the same low-pass filter as the previous map. The correction is made with the density of 2600 kg/m³. The map essentially displays a gravity depression, about 75 milligal deep, roughly reflecting the topography above sea level. But in this there is a bit of a paradox!

The average height of the landscape in the middle of Iceland is about 1000 meters. Assuming that the landscape was in perfect buoyant equilibrium - the equilibrium plane was level at sea level - then the density of the rock could only be about 1800 kg/m³ to match the 75 milligal Bouguer anomaly! The explanation is, of course, what is stated above: The equilibrium surface is not smooth, but rises up to 300 metres. The height of the landscape to be corrected for is therefore much lower than the altitude suggests and this corresponds well with a rock density close to 2600 kg/m³.

Figure 5. The imaging model for the seismic velocity

At the time when we were pondering the picture that the gravitational field represents of Iceland and what lies beneath it, we came across a remarkable paper by Kristján Tryggvason et al. where delays in the arrival time of seismic waves from distant earthquakes are used to calculate variations in the seismic velocity below Iceland to a depth of nearly 400 km.^*

The article sets up a mathematical model of the Earth's crust and the upper part of the mantle beneath Iceland. The mathematical model extends from 11° to 26° west longitude, from 61.8° to 67.9° north latitude, from the earth's surface to a depth of 375 km. This box is divided into 6×6×4 smaller blocks and the trajectories of all observed seismic waves are traced through the blocks. In this way, a rough picture (6×6 blocks) is obtained of the variations in seismic velocity in four depth intervals. The top layer extends down to a depth of 75 km and corresponds approximately to the lithosphere. The other three are each 100 km thick and extend down to a total depth of 375 km.

It should be noted that the following speculations, comparing the results of Krisján Tryggvason et al. with other data, arose without their involvement. What may be wrong is therefore entirely our fault.

* Kristján Tryggvason, Eystein S. Husebye og Ragnar Stefánsson 1983. Seismic image of the hypothesized Icelandic hot spot. Tectonopysics 100, 97–118.

Figure 6. Seismic velocity in the lithosphere and fissure swarms

The top layer of the imaging model, which corresponds approximately to the lithosphere, contains a low-velocity zone that coincides with part of the active volcanic zone. Figure 6 shows how the main fissure swarms associated with central volcanoes* and the lithospheric low-velocity anomaly go together. The seismic anomaly is shown with a -2% equivalent line, which represents a 2 % decrease in velocity within the restricted area. Specifically, it takes seismic waves over 2% longer to travel through the lithosphere in this low-velocity region than the average seismic velocity in the layer predicts.

The low-speed area stretches from the Tjörnes fracture zone in the north towards the Vatnajökull glacier, turns there to the southwest and includes both Hofsjökull and Langjökull. The velocity anomaly is greatest at the turn, where the SV-NA and N-S directions meet. In fact, one might say that in the low-speed region the lithosphere appears missing, similar to what is seen at oceanic ridges where crustal rifting takes place.

* Páll Einarsson og Kristján Sæmundsson 1987. Earthquake epicentres 1982-1985 and volcanic systems in Iceland: A map. Í: Í hlutarins eðli: afmælisrit til heiðurs Þorbirni Sigurgeirssyni prófessor. Menningarsjóður.

Figure 7. Seismic velocity in the asthenosphere and
the uplifted equilibrium surface

The second top layer of the imaging model spans a depth range of 75-175 km, which is the top of the asthenosphere. At this depth there is a low-speed area that coincides with the main anomalies in the free-air gravity map (figure 2). The map in Figure 7 shows how these two phenomena go together. The low-speed area is represented by an equivalence line of 0 %. Within it, the arrival of seismic waves from distant quakes is delayed compared to the average for this layer in the model.

The anomalies in the free-air gravity map are caused by the uplift of the equilibrium surface for the buoyant landscape. The cause of this uplift is presumably found in the mantle - the material underlying the uplift is presumably lighter than other mantle material beneath the land. The correspondence with the low-velocity region at depths of 75-175 km suggests that this lighter mantle material is largely present there.

Next below, at a depth of 175-275 km, there is no low-velocity area at this location. This suggests that the anomaly in the mantle occurs within a limited depth range. However, the resolution in the scan is not high, so the depth scales in the model must not be taken too literally.

Figure 8. Seismic velocity in the mantle and floating landscape

The bottom layer of the imaging model spans the depth range of 275-375 km, which is roughly the bottom of the upper mantle. Below that there are rapid changes in density thought to be caused by the transformation of minerals such as pyroxene and olivine. This layer contains an extensive low-velocity area that coincides with the landscape in buoyant equilibrium (Figure 3). The map in Figure 8 shows how the two go together. The low speed is represented by both 0 % and -2 % contours.

It is tempting to think of these two phenomena as reflections of the mantle plume beneath Iceland. The relatively hot and light material rising slowly in the mantle plume is reflected in the regular shape of the floating crust and the lowest seismic velocity is observed around the center of the hotspot. Then, at a depth of 150-200 km, the pressure would have dropped enough for this hot material to partially melt, resulting in the anomalies that raise the buoyancy plane and are reflected in the free-air gravity map (figure 2).